by Corry Shores
[Search Blog Here. Index tabs are found at the bottom of the left column.]
[Central Entry Directory]
[Jakob von Uexküll, entry directory]
[Uexküll, Theoretical Biology, entry directory]
[The following is summary. All boldface and bracketed commentary is my own. Proofreading is incomplete, so please forgive my typos. Page citations refer to the 1928 German edition first and to the 1926 English edition second. Note for this section: I do not yet have the original 1920 German first edition on which the translation seems to have been based. So some references to prior sections will refer just to the English edition for now.]
Summary of
Jakob von Uexküll
Theoretical Biology
[Theoretische Biologie]
Ch.3 The Content-Qualities
[Die Inhaltsqualitäten]
3.2 The Form of the Quality-Circles
[Die Form der Qualitätskreise]
Brief summary:
We obtain sense data from various nerves, along with local signs telling us the spatial location of the stimulus and as well moment-signs telling us the temporal location. For each location there can only be one quality datum, or “content-quality”, and thus there cannot be contradictory qualities in the same location. The perceived space around us forms spheres of larger sizes, with us at the center. So we can think of there being content-qualities occupying spatial locations along a spatial circle haloing around us. But qualities can also take on circular relations of intensity. This is evident with the quality circle for color. As we move from red to yellow, there is between them a continuum of color mixture. From yellow we go to green, but since green is qualitatively very different from the red-yellow spectrum, we can think of the yellow-green continuum as moving in another direction. We turn yet another direction to go from green to blue. What comes after blue in the color spectrum is violet, which is a mixture of red and blue. Thus we turn from blue back toward red to complete our circle.
Summary
§369
[In chapter 1 we noted that the animal’s perception of space is like a series of larger and larger spheres centered on the creature.]
[The first couple paragraphs of this section will summarize some ideas from prior sections. Not all of the prior sections correspond between this English translation and the German edition I am using, presumably because the English edition is based on the German first edition, which I do not currently have access to. As such, I will refer to the English pages primarily to summarize these points. Uexküll begins this section by saying that in order to investigate the appearance-worlds [Erscheinungswelten] of others, we need to have an exact knowledge of our own (62 / 72). He then refers to an idea from the first chapter on space. One notion from the section “The Space of Animals / Die Räume der Tiere” (36 / 40) is that we can think of animals having a sphere of space surrounding them that is “like an invisible soap-bubble” (62 / 72). In this earlier section, he addresses the question, ‘What does space look like that surrounds animals?’ (??? / 40). We first need to create a diagram for human space, and then we modify it “according to the faculties of the animal under investigation” (??? / 40). To do this we should first note the idea of local signs. The basic idea is that our nerve endings give us qualities, like touch sensations. But they also tell us where on the body the sensation is coming from. These spatial indicators are called local signs [Lokalzeichen] (5 / 2). (See their description by Lotze here. The basic idea in that context is that the sense data coming from the nerves is not enough to provide spatial relations between the data. And thus we would not know how to spatially arrange the data in our minds. What is also needed are the local signs which give us those spatialized relations between impulse sources.) I am not certain, but I get the sense that the local signs are indicative not simply of the bodily location of the nervous stimulus but as well can be indicative of spatial features of the stimulus source out in the world. So perhaps for example there are local signs to tell us when a visual stimulus comes more from the distance, but I am not sure. “Direction-signs” involve the relation between local signs. “The sensation of direction comes in only when one local sign strikes up while the others most nearly related to it have not yet quite died away [...]. [...] what we call ‘motion’ is only that change in quality which is connected with the quality of direction.” (7 / 6) “I call that which connects local signs a direction sign” (???{cf.11} / 6). (In other words, if a tactile stimulus moves from one nerve on our skin to another located somewhere else on our skin, the relation between the local sign for the beginning place and that of the ending place gives us a direction of the movement of the stimulus). We return now to the space of animals and humans. Uexküll has us consider there being three circular pieces of cardboard, and we intersect them along the three planes of direction (??? /40-41). (I suppose we have one lying flat horizontally. The other two will stand upright vertically. So the second of the three circles will be vertical facing forward, while the third will be vertical at a right angle to the second, with all three of them sharing the same center-point. The three circles give us the main directions: forward-backward, up-down, and right-left.) We then place spheres of increasing size emanating from the center-point. There will be small spheres near the center that are placed close to one another. As we move outward to larger spheres, the distance between them increases. This distance between the spheres represents the discernible layers of depth. We are most sensitive to the distances in front of us, so we can differentiate the layers more precisely. This is why the spheres nearest us are closer together. But as we go further away, we are less able to make such precise determinations of distance. And, “Each sphere has just as many places on it as there are local signs available. Hence there are much fewer to the same surface of the larger and more distant spheres than there are on the smaller ones close at hand” (??? / 41). (The idea here might be that since the only way we have to differentiate spatial properties of the stimuli is by means of local signs, then there are just as many locations in the sphere as there are local signs for distinguishing them. Now if we consider geometrically how the rays of an angle grow further apart as they move into the distance, then we can imagine that the locations on the more distant spheres are also spaced further apart. “Place” is like all the locations on each spherical surface along one ray, standing one behind the other. “Position” is the location upon one such sphere. Position might instead just be like a designation of the sphere itself, which makes a coordinate with the place, or it might be that coordinate itself. I am not sure. The next idea might be that we can also transpose the places in our perception with places of the world understood according to more of a homogeneously metrical, decentralized understanding of space, as it is understood objectively in physics.) But space as we experience it, or “intuited space,” is what concerns us in biology (??? / 42). Humans have the three planes of direction (the three cardboard circles), but other animals may have just two or one. They may even have none and instead work only with the line of the horizon. For humans the larger and larger spheres are related also to our motor behaviors and thus we can use them to think of steps-into-distance. When studying other animals we should also look at their capacities for making steps-into-distance with respect to their spatial perception. We also must learn the number and distribution of the places in the animals space. (??? / 42). The next idea in this earlier section takes us to the notion of the soap bubble which is relevant to the current section we are examining. The idea seems to be that there is something like an a priori sort of representation of space in animals in the sense that the creature has a certain outer limit to their perception of space, but they assume there is more space beyond that boundary and that it will become apparent upon moving toward it. In this way, we can think of each animal being surrounded by a “soap-bubble” into which parts of the world enter as it moves its bubble around.
Every spatial animal world, however limited as regards places and steps-into-distance, and even though it be without planes of direction, is nevertheless surrounded by the pure extended, which, as necessary form, precedes all space-creating. The extended lies immediately behind the last step-into-distance. So the space peculiar to each animal, wherever that animal may be, can be compared to a soap-bubble which completely surrounds the creature at a greater or less distance. The soap-bubble of the extended constitutes for the animal the limit of what for it is finite, and therewith the limit of its world; what lies behind that is hidden in infinity.(??? / 42)
So returning again to the current section, Uexküll says that we can describe the space around an animal as being like an invisible soap-bubble [Seifenblase] (62 / 72). After reminding us of a few features of animal space, he notes that “The laws of the content-qualities of our mind are as changeless as the spatial laws of our appearance-world [Erscheinungswelt]” (63 / 72, bracketed insertion mine. See pages 22-23 / 14-16).]
A preliminary condition for the investigation of the appearance-worlds of others is an exact knowledge of our own. In the first chapter, which dealt with the spatial qualities, we succeeded in forming an idea of space that permitted us to describe around every animal a space like an invisible soap-bubble, within which all its activities were carried on. A number of fixed places give support for its sense-organs, and a definite number of direction-steps give the measurement of the magnitudes, and determine the movement of its limbs. The direction of movement is fixed, in many cases, by immutable direction-planes. The laws of the content-qualities of our mind are as changeless as the spatial laws of our appearance-world [Erscheinungswelt].(62-63 / 72, bracketed insertion mine)
§370
[We will study the ways that the content-qualities work in our minds by seeing them in action.]
Uexküll, again referring to past sections, says that we cannot simply learn the laws that regulate our own mind, because “The activity of our qualities consists in constructing our appearance-world [Erscheinungswelt]” (63 / 72, bracketed insertion mine). [The idea seems to be that the laws of the organization of the qualities given to our senses only come to light when we see that organizational process. Let me quote.] “Considered by themselves, all our qualities seem just a confused heap of building-materials, all more or less alike. The laws are revealed only when the work of construction is in progress” (63 / 72).
§371
[Content-qualities are found at particular locations by means of local signs. The same place cannot have contradictory content-qualities.]
[The next idea seems to be that the content-qualities coordinate with spatial locations by means of the local signs. Perhaps we might consider looking at a painting. Different spatial locations (corresponding perhaps somehow to different optical nerve locations) give us different color qualities. What is important to emphasize here is that for each location there can only be one content-quality for each sense modality. In other words, one location cannot have contradictory qualities.]
When the content-qualities are fitted into the local signs, fixed places appear, having definite properties. And now the outline of a fundamental law is revealed. The “circles” of relationship, which were but faintly indicated in the original material, can be de-limited one from another. Each place, that is to say, can receive only one property from each quality-circle. A certain place may be blue-green, but never blue | and green. It may be of medium hardness, but never both hard and soft; it may be lukewarm, but never both hot and cold at the same time.(63 / 72-73)
[I am not sure I understand the idea of the quality-circles. Given that they are related to local sigs, perhaps they would be like qualities located on one spatial sphere. Or perhaps the circle is meant simply to give a non-spatial sort of indication of relations. I am not certain.]
§372
[Given that there can only be one content-quality per place, that means you cannot have two different related qualities in the same place.]
[The next idea seems to reinforce the prior one regarding there being just one content-quality per place. Now the notion emphasizes that the appearance of a quality at one place prevents there being a related quality at the same place. Let me quote to be sure:]
This circumstance (i.e. that at each place properties from all the relationship circles may be assembled, but never more than one single quality from each) shows that the qualities of each circle are connected together by law in such a way that the appearance of one quality excludes the simultaneous appearance of a related quality at the same place.(63/73)
§373
[We can consider the content-qualities extensively as occupying spatially a place in an extensive quality-circle, or we can think of content-qualities occupying an intensive quality-circle.]
[The next idea seems to be the following, but since I am uncertain, please consult the quotation to follow. We so far have considered the extensive spatial relations between the quality contents, as their spatial relations are given by means of the local signs. There is also the matter of intensive differences between the content qualities. We will now form intensive quality-circles so to study their laws. Let me quote, as there is more to this that I am missing:]
As soon as it enters into activity, the material of our mental qualities reveals a form governed by law, which form may enter into appearance along with space and time, or must especially be sought out for the content-qualities. Since only the form of the extensive quality-circles of the local signs and direction-signs is given by intuition, we must try to include within that extensive form the intensive quality-circles, in order to arrive at a clear idea of the laws governing them. To admit of comparison between the extensive qualities themselves, we have already expressed in spatial terms the form of the moment-signs, which is not intuited; and so we shall now attempt to represent in terms of space the other quality-circles likewise.(63 / 73)
§374
[We can arrange the relations between colors as being on a spectrum but one that wraps around upon itself. The color mixtures can take a hexagonal form.]
Uexküll has us consider if we view a color spectrum. [Let us consider this manner of presenting one:
] Uexküll says when we look at it, “four fixed points immediately strike our eye, at which certain colours emerge pure from the mixture,” namely, pure yellow, red, green, and blue. But between every two points of pure color there is a continuum of color mixture. So we begin with two, the red and the yellow. We will now make our own diagram for these color quality relations. We have fixed yellow and red as points of pure color.
And we can draw a straight line between them to represent the continuum of mixture between them (with a color-band added in the diagram below).
[The next observation is interesting. Uexküll seems to be saying that the difference between these two color points varies continuously and linearly. But as we go to a third color, it is not on that same continuum of variation. He does not explain why, but it seems that it has something to do with the qualitative difference that defies quantitative variations of more and less. In other words, we can say that between yellow and red is a range of variations of yellow-red mixtures, but when we move further to green, there is not a continuation that carries forward a larger range of red-green mixtures (As I understand, if you mix red and green light, you get yellow. But maybe the idea here is that we do not perceive yellow as somehow being between green and red, although we perceive orange as being between yellow and red, because it seems to have qualities of both). Rather, we are starting a new mixture sequence, and for this reason he depicts the variations as taking a right turn.]
We cannot carry the straight line from the red any further, for here we are dealing no longer with red-yellow colours, but with colours that are a mixture of yellow and green. To express the difference, we must give the line a new direction. So we shall place the green point below the yellow and connect these two by means of a line representing the colours that are a mixture of yellow and green.(64 / 74)
This holds again for the next sequence between green and blue.
At this point Uexküll makes another interesting observation, namely, that as we go further from blue in the linear spectrum, we move into violet, which is a mixture of blue and red. This brings us then back to red.
As we can see from this wheel portrayal, the spectrum can be understood as circular.
Uexküll describes further modifications:
So we may bring back the line from the blue point straight to the red. In this way we get a square, on which we may set equilateral pyramids, one above and one below; this gives a hexagon. The apex of the upper pyramid shall be white and that of the lower one black. While all the angles of the hexagon carry pure colours, on each of the edges will lie colours arising from a mixture of two. The faces of the hexagon, on the other hand, will display colours formed by mixture of three colours, which radiate outwards, in diminishing strength, from the three angles surrounding each face. If we make a transverse section through a face, we can always tell at what level the single colours lie above one another, in order to produce in this way all possible mixed colours.(64 / 74)
[I can imagine combining two pyramids with a square base to them, placing them base-to-base, so to form an eight-sided diamond-like figure. But I do not know how to obtain a hexagon from that. Perhaps the “equilateral pyramids” are triangular on all faces, and perhaps furthermore they are placed together offset so that their bases taken together create the six points of a hexagon. So it might perhaps resemble something along the lines of this diagram.
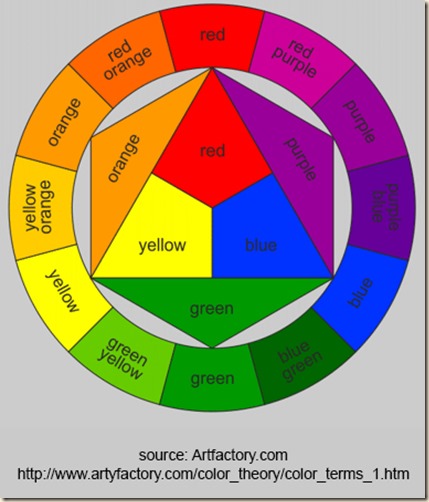
(And possibly furthermore we are to think of the top pyramid having pure tones at its base, but tapering continuously to white at the apex, and the bottom one tapering to black. I am not sure however why they would converge at a point. Maybe the top pyramid represents the combination of colors as light and the bottom as the combination of colors as pigments. If it were just to show light and dark variations of each color hue, I would think they would not converge at a center but rather rise up and down like in a cylinder.) But in that case of combining pyramids with triangular bases, I am not sure how to situate them in relation to the original square we drew. So I am not certain about the shape Uexküll is forming.]
§375
[This hexagonal arrangement provides a visualization of the laws of the color-quality circle.]
This color hexagon depicts the laws in the circle of the color-qualities (64 / 74). [The laws seem to be the laws of color relations, including those of mixing.]
Works cited (in this order):
Uexküll, Jakob von. 1928. Theoretische Biologie, 2. gänzlich neu bearbeitete Auflage. Berlin: Springer.
Uexküll, Jakob von. 1926. Theoretical Biology. Translated by Doris Livingston MacKinnon. London: Kegan Paul, Trench, Trubner & Co. / New York: Harcourt, Brace & Company. PDF available at:
http://www.archive.org/details/theoreticalbiolo00uexk
Other image sources:
Visible color spectrum, linear:
SOHO (Solar and Helospheric Observatory). “Making your cerealbox spectroscope.” https://soho.nascom.nasa.gov/classroom/spectroscope.html
Color spectrum wheel:
Programming Design Systems. “Computational Color”. http://printingcode.runemadsen.com/lecture-color/
Color wheel with hexagon:
Artfactory.com. “Color Terms for Art and Design, I”.
http://www.artyfactory.com/color_theory/color_terms_1.htm
.
No comments:
Post a Comment