The DeComposition of Our Body's Functions. Review and philosophical application of Compound Functions in Edwards & Penney's Calculus (Section 1.4)

presentation of Edwards & Penney's work, by Corry Shores
[Search Blog Here. Index-tags are found on the bottom of the left column.]

[Central Entry Directory]
[Mathematics, Calculus, Geometry, Entry Directory]
[Calculus Entry Directory]
[Edwards & Penney, Entry Directory]

Edwards & Penney's Calculus is an incredibly-impressive, comprehensive, and understandable book. I highly recommend it.

[Search Blog Here. Index-tags are found on the bottom of the left column.]

[I your author am not a mathematician; I am merely an admirer of Edwards & Penney's wonderful calculus book. Please consult the text or other references to be certain about anything in the summary below. I mean this emphatically.]

[Other Entries in the Edwards & Penney's Calculus Series]

The DeComposition of Our Body's Functions

Review and philosophical application of
Edwards & Penney's Calculus
Compound Functions
in
Section 1: Functions, Graphs, and Models
Subsection 4: Transcendental Functions

What Do Compound Functions Got to Do With You?

Consider if we are trying to lose weight. So we go on a diet. Over the course of a day, we begin to feel our appetite increase. So this would be one function: as time increases, our appetite increases. But then, as our appetite increases, there is a decrease in our willpower to hold strictly to the diet. So our decrease in willpower is like a function compounded on the first function. Our lives are filled with many such highly complex functional relations.

Brief Summary:

Functions can be compounded one-upon-another as if the output of one machine instantly becomes the input of another.

Points Relative to Deleuze
[Under Ongoing Revision]

We might find a composition of functions in Deleuze's account of sensation, building from his portrayal of Spinoza's affection. Deleuze's example is that we are looking for our glasses in a dark room. Someone enters and turns on the light. This causes us to make a transition from the way we are affected by the dark to the way we are affected by the light. Because we are searching for our glasses, this affection increases our power to act. Consider instead if we are meditating in the dark room. Then when someone turns on the light, this dazzles us and ruins our concentration, and we lose the power to act.

Affection for Spinoza is a matter of our body's composition. What gives our body its power is the differential relations between the speeds of its simple bodies. But its simple bodies are like calculus differentials, infinitely small parts that only obtain a finite value when put into a differential relation with other simple bodies. Consider if we take poison. This will disrupt the ways the simple bodies in our body differentially relate to one another. This is why we die. Our bodies no longer express the range of differential variations that define us. Instead, they eventually express for example dirt.

Now, Deleuze also places a value on our internal decomposition when it comes to the sensations that affect us. The discord of our faculties is what makes sensations more intense [see here and here.] Also consider Spinoza's Ethics Part IV, Proposition 39, Scholium. He writes:

It sometimes happens, that a man undergoes such changes, that I should hardly call him the same. As I have heard tell of a certain Spanish poet, who had been seized with sickness, and though he recovered therefrom yet remained so oblivious of his past life, that he would not believe the plays and tragedies he had written to be his own : indeed, he might have been taken for a grown-up child, if he had also forgotten his native tongue. If this instance seems incredible, what shall we say of infants? A man of ripe age deems their nature so unlike his own, that he can only be persuaded that he too has been an infant by the analogy of other men. (Elwes translation)

From what Spinoza says about the decomposition of the body, it would seem that to change from child to man, or from poet to someone different, would be a decomposition, and thus a loss of power. But could it be that Deleuze takes the opposite view? To change from child to man is not an expression of our body's frailty. It rather shows our body's power to become something new. Imagine we get a sickness like the Spanish poet, and we no longer recognize our past selves. This would seem to be a loss of power. But that would only be so when we take the perspective of the self whom our body no longer expresses. But our bodies are always as perfect as they can be, given the conditions affecting them at any given moment. So to become someone else is not really a deprivation. It could be a testament to the power of our bodies to become new things, to express new essences. The question for Deleuze might not so much be "What can a body do?" as much as it might be "What can a body become?"

So here would be the composition of functions in this case. A certain affection might for Deleuze increase the intensity of sensation. But that means a decrease in the organization of our faculties and our body's compositional relations. Yet this again would be an increase in our body's power to become new things, to express new relations and essences. So it ultimately is an increase in our body's expressive power.

Edwards & Penney's Calculus
Compound Functions
in
Section 1: Functions, Graphs, and Models
Subsection 4: Transcendental Functions

Recall the function machine.

We can think of functions like these 'black box' machines. The machine takes-in a number from one set of values, and it gives-out a value from another set. So consider the squaring function:

The machine takes-in a 2, for example, and then gives-out a 4.

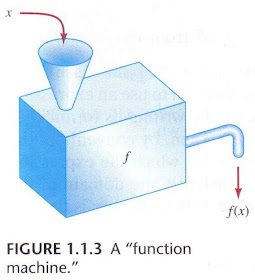
We can also compound our functions. So we can take the result of one function, and then put it into another 'function machine', if you will.

So let's begin with the function g(x). We put in x, and it gives-out u. We then have another function f(u), which is the same as f(g(x)), and we read it as, 'f of g of x'. So we plug the u into the f function, and it gives out the value for f(u). We can then call f(u), or f(g(x)) a new name. We can call it h(x). So,

h(x) = f(g(x))

This requires that all the x values in g's domain correspond (by means of g) to values in f's domain. We call the g function here the 'inner function', and the f function would then be the 'outer function' in this case.

Edwards & Penney now give an example that will illustrate something about notation for composition of functions.

We will first find f(g(x)). First note the g(x). If our number for x is larger than 1, then we will have a negative number. But when we apply it to the f function, we will have the square root of a negative number. So instead we qualify it:

But let's instead consider if we first take f(x), and then after that apply it to the g function. To avoid having the square root of a negative number, we must first begin with a positive. Then after that, there are no restrictions for the g function. So

Edwards and Penney would like then to distinguish two notations:

f(g(x))

and

f ○ g

Our example above shows that the composition of functions f and g is not the same as g and f.

f ○ g ≠ g ○ f

So the notation f(g(x)) might remind us of multiplication notation. In the case of multiplication, it does not matter what order the functions are multiplied. But as we see in the composition of functions, the order does matter sometimes.

from Edwards & Penney: Calculus. New Jersey: Prentice Hall, 2002, pp. 36-37.

Spinoza. Ethics. Transl. Elwes. available online at:
http://ebooks.adelaide.edu.au/s/spinoza/benedict/ethics/index.html