by Corry Shores
[The following summarizes part of chapter 2 in Bergson's Duration and Simultaneity. Paragraph headings are my own. My personal commentary is in brackets.]
Relations of Durations
Henri Bergson
Duration and Simultaneity
Ch. 2. Complete Relativity
Previously Bergson explained the Michelson-Morley experiment. When objects move, time dilates and space contracts. He showed us the formulae we might use to rectify our measurements so that they indicate they way they were from the perspective of a motionless ether.
§24 Our New Relation to Einstein's Relativity
So we might think that there is a motionless frame of reference to which all moving reference frames may be calibrated. Under that view, we would be dealing with a "unilateral relativity." (19b). In Einstein's theory of reciprocal relativity, we still use the same equations that adjust for the contraction of moving bodies, the expansion of their times, and the breakup of simultaneities into successions, except now all these changes are reciprocal phenomena. To see all motions relative to a fixed frame would be to regard things in terms of "single relativity." But to see them reciprocally would mean we are dealing with a "double relativity." Bergson explains that we need a theory of single relativity before we can move-on to a theory of double relativity. Consider when we see everything as reciprocal. There may still be a system that is motionless relative to the others, even if it is not somehow absolutely motionless. The mathematics will work out the same whether the system is relatively motionless or absolutely motionless. But things are not the same for the philosophy behind each theory. "For if S is at absolute rest and all other systems are in absolute motion, the theory of relativity will actually imply the existence of multiple times, all on the same footing and all real. But if, on the other hand, we subscribe to Einstein's theory, the multiple times will remain; but there will never be more than a single real one among them, as we propose to demonstrate; the others will be mathematical fictions" (20b). For this reason, Bergson believes that Einstein's theory will resolve the philosophical difficulties involved in these problems. We need now discover how to understand the "distortion of bodies," the "slowing of time," and the "rupture of simultaneity" in Einstein's theory. Then, we will look back at the previously theory to see that it was a necessary starting point.
§25 The Reciprocity of Relativity
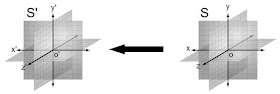
Recall our system S and S' illustration.
System S is at rest in the motionless ether, while system S' moves away from system S. Yet, no one has ever perceived the ether. It is a theoretical construct propped into physics calculations. Nonetheless, we do observe such systems as S' moving away from other systems that seem motionless in relation. And unless someone proves otherwise, we must assume that light remains the same speed, no matter if it is moving with a speeding or still system.
Bergson returns to our three previous assertions.
1. S' shifts with respect to S.
2. Light has the same speed in both systems.
3. S is stationed in a motionless ether.
The first two assertions are facts. But the third is a hypothesis. So let's now reject the hypothesis. All we have left are two facts. Yet, when we remove the third assertion, we must alter the first one. We could also have said that S shifts relative so S'. Hence we now have reciprocity of displacement. All we really perceive are the changes in distance between bodies, and not which ones are unmoving. We might just say that the distance between bodies increases, or that they both move with respect to one another. "The 'reciprocity' of motion is therefore a fact of observation" (21b).
§26 The Philosophy of Reciprocity
Nonetheless, we often perceive things as though one thing is motionless and the other mobile. We might think of someone jumping. We do not think that the jumper and the earth move further apart from each other. Rather, we think that the jumper moves away from the stable earth. Hence we do not always perceive reciprocal relativity, especially when it concerns movements that we perform out of our own volition. Science conceives space and time as homogeneous media. But philosophers who study the nature of their actions can regard there being non-reciprocal motion.
§27 Relativity's Absolute Historical Change
Descartes posed a radical relativity of motion. Science presumably also takes-up such a position, but only "hesitantly and incompletely" (22d). For example, physicists might assume reciprocal relativity when dealing with constant motion, but they needed to put it aside when dealing with acceleration. And by putting aside Descartes' radical relativity, physicists were able to introduce the principle of force. They did so by "carving out and isolating parts within the whole" (23c). And they found that the centrifugal forces in rotational motion "seemed to attest that one was now dealing with a true absolute; and that all other accelerated motion was equally to be considered absolute" (23d). This theory held until Einstein. But "No philosopher could be entirely satisfied with a theory that regarded mobility as an ordinary relation of reciprocity in the case of uniform motion, and as a reality immanent in a moving body in the case of accelerated motion" (23-24). Yet now [with Einstein's theory] we may say that every motion is relative [accelerated and otherwise]. It is for this reason that the general theory of relativity marked a significant change in the history of ideas.
§28 What's So Special About the General Theory?
Einstein also had a special theory of relativity. It reflected on time and simultaneity and was concerned with uniform motion. Yet this special theory also declares motion to be reciprocal. So it evokes the general theory as well.
§29 We are Free to Be Motionless
So let's take-up this assumption that all motion is relative. That means there is no absolute point of reference and no privileged system. Hence an observer inside a system will have no way to know if her system is in motion or if it is at rest. In fact, the question has no meaning when we suppose radical relativity. In fact, she is at liberty to say her system is motionless, and thereby make it the system of reference.
§30 Relevant Questions of Relativity
According to Bergson, "What is immediately given to our perception, we explained, is a continuity of extension upon which qualities are deployed; more especially, it is a visual continuity of extension, and, therefore, of color" (25a). Science supposes there is some physical basis for colors. So even if our eyes were structured differently, and saw colors differently, they still would be perceiving the same physical conditions, only in a different way. Hence when we "speak only of a qualified and qualitatively modified continuity, such as color and color-changing extension, we immediately express what we perceive, without interposed human convention--we have no reason to suppose that we are not here in the presence of reality itself" (25b). We consider appearances to be real unless they are proven otherwise. Matter, then, is immediately perceived as a reality. But what about the individual things we see? It seems arbitrary how we cut-up the world around us. Perhaps other species do it in a radically different way. "It dissolves the body into a virtually infinite number of elementary corpuscles; and, at the same time, it shows us this body linked to other bodies by thousands of reciprocal actions and reactions. It thus introduces so much discontinuity into it, and, on the other hand, establishes between it and the rest of things so much continuity that we can gather what there must be of the artificial and conventional in our division of matter into bodies" (25d). Yet, wonders Bergson, does the same not hold for motion? When we see something move, can we really distinguish its movement as being independent of all movements it relates to? Also consider color. Presumably it can result from vibratory oscillations of matter. Such motion is relative. But the light it propagates is not. So, Bergson wonders, should we still consider these motions to be relative? And light's motion is not relative. But then how do we relate it to moving systems? We speak both of light's motion and as well we speak of the motion of physical bodies. But the motion of light is non-relative, while the motion of bodies is relative. So does the word "motion" have the same meaning in both cases, if their motions are so radically different?
§31 What 'Systems of Reference' Refers to
Bergson now has us assume the reciprocal relativity position. We used certain terms previously, but now they must be redefined. First recall how the x, y, and z axes described three dimensional space.
Bergson defines the term "system of reference" as being a trièdre trirectangle "with respect to which we shall agree to situate, by indicating their respective distances from its three faces all points in the universe" (26).
A scientist resides at the vertex of the trihedral trirectangle, which serves as his observatory of the whole cosmos. This system of reference remains motionless, all while its reference points are being used. But recall that we have done away with the ether. That means his system of reference is the standard one only because we have arbitrarily decided that it is motionless in relation to the others. We just as easily could have selected some other system and called it motionless, which would make the scientist's one the system that is in motion. Now, we are saying that the second system is not in motion, but the first one is in motion. We could then if we wished selected a third system, and called it motionless. That would make both the first and the second one now have motion, in relation to the motionless third one. However, Bergson says that we can conceive of the second one as being in motion even without positing a third motionless system. [Perhaps this requires that for one instant we see the first system as immobile and thus the second as mobile. Then in the next instant we see the second system as immobile and thus the first as mobile. Through these tiny but rapid steps, both systems can be conceived as having motion. Bergson writes, ] "It is true that this second system can in turn be mentally set in motion without thought necessarily electing to settle in a third system. But in that case it oscillates between the two, immobilizing them by turns through goings and comings so rapid that it entertains the illusion of leaving them both in motion. It is in this precise sense that we shall speak of a 'system of reference' " (27b). "Mais alors elle oscille entre les deux, les immobilisant tour à tour par des allées et venues si rapides qu'elle peut se donner l'illusion de les laisser en mouvement l'un et l'autre. C'est dans ce sens précis que nous parlerons d'un « système de référence »." (50).
§31 The Ground is Constant
Now consider the events on earth for example. Many events transpire on and below its surface. But we consider all together as though they were part of a stable system of reference, the earth. Bergson calls such a system a "constant system" or just simply "system." This term applies to "every group of points which retain the same relative positions and which are therefore motionless with respect to one another" (27).
§32 Our Freedom to Be Fixed in One Place
It is possible that a constant system can serve as a system of reference. This happens when we arbitrarily designate one as such. The reference system will have its trihedral vertex.

§32 If Everything is in Relative Motion, then Everything is Motionless
[Now, let's imagine that we have many moving systems, with one system of reference. If we extend that one system out indefinitely, it will engulf all the rest, hence placing everything in the same motionless system of reference.] Consider if we could have a clock at every point in space. Each one would be its own vertex of the trihedral. Hence, writes Bergson, "the transition from 'system' to 'system of reference' will be continuous if we take the position of the theory of relativity" (27).
§33 Let's Move On Now
Our previous efforts to define our terms will allow us to further evaluate Einstein's theory.
Bergson, Henri. Duration and Simultaneity. Ed. Robin Durie. Transl. Mark Lewis and Robin Durie. Manchester: Clinamen Press, 1999.
The original French version is available online at:
Images from:


No comments:
Post a Comment